三角锥上的数字
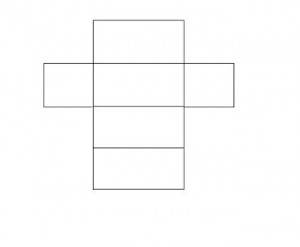
The Problem A tetrahedron (as shown below) has four sides and six edges. Numbers from 1 to 11, except number 10, are each assigned either to a vertex or to an edge. The number assigned to an edge must equal to the sum of the numbers assigned to the two endpoints. Each of the numbers must be used exactly once.
问题 一个四面体有 4 个顶点和 6 条边。从 1 – 11 的数 （没有10），一一地被分配到边和顶点。每条边上的数字是该边两个端点的数字之和。上述每个数字必须刚好使用一次。
Note: Another name for the tetrahedron (四面体) is triangular pyramid (三角锥).
Can you find a solution that satisfies ALL of the above conditions?Let’s work on this problem until we find a solution!
第一步总是最难的。要大胆地试！
First step is always the most challenging, as we are usually not sure which path to take when facing a difficult problem. Don’t be afraid to try; when it does not lead to where we expect, reflect on why it does not work and have another try where we have a better chance.
（第一步）试了几次后，发现最小的两个数1 和 2应该被分配到顶点而不是边上。在连接1，2的边上的数字应是3。
(Stage 1) After a few trial-and-errors (or guess-and-checks), we come to focus on the two smallest numbers 1 and 2, and decide that they must be assigned to the vertices, not the edges.
Why? Because if one of them (say 1) is assigned to an edge, they must be the sum of two numbers assigned to its two endpoints, therefore the two numbers at the endpoints must be even smaller. However, this is impossible, as the number 1 and 2 are already the smallest.
Which vertices to assigned numbers ‘1’ & ‘2’? In a tetrahedron, any two vertices are adjacent, and the whole solid is highly symmetric – by rotating /reflecting, any two adjacent vertices can be transformed to the two designated ones. This is settled! We can assign numbers ‘1’ & ‘2’ to any two vertices, and the edge connecting those two vertices shall be assigned number ‘3’.
Well begun, half done! But there is other half, for which we start by some frantic trying.
（第二步） 最大的数字11 应该分配到一边上。 这条边的端点不是 1 或者2。
(Stage 2) This time we look at the largest number 11. Suppose we assign it to an edge that’s adjacent to ‘2’. For the edge, one endpoint is ‘2’, the other endpoint – it must be ‘9’. We are now in a situation that we cannot go on any further. Con
sider any edge with endpoint ‘9’ (other than the edge we already assigned ’11’): which number shall we assign? It can neither be ‘1’ nor ‘2’ nor ‘3’ (since all these numbers have been used, we are not supposing to repeat numbers). Smallest available number is ‘4’, but 9 + 4 = 13, which is not in the given set of numbers.
So the edge to which number ’11’ is assigned cannot have any endpoint ‘1’ or ‘2’; and take another look at the figure, we decided that it must be the edge that is the furthest from the edge ‘3’. And we mark 11 there.
(第三步） 分解数字 11 成两数之和。11 = 4 + 7 = 5 + 6. （注意，数字 1，2，3 已被用过了。） 只有这几种可能性，试试就得了。
(Stage 3) 
Decompose 11 into the sum of two positive numbers (but without using number ‘1’ ‘2’ ‘3’), we have the following two: 11 = 4 + 7 = 5 + 6. A couple of trials will finally lead to the solution! We leave it to be worked out by the reader.
What knowledge we have used in this whole process?
（解决本题时用到了什么？）用到了数和运算，用到了形状（点对和连接的线段），加上一些逻辑推演。所以这个问题是综合性的呀！
还有，从图形的对称性，大大减少要考虑的情况。
- Numbers and its operation – you have to understand addition and subtractions of integers.
- Shapes – you are not asked to do any calculation like perimeters and areas, but you have to understand an edge is constituted with two endpoints, and which edges meet and which do not (we call them the furthest edges).
- Logic deduction – see how we’ve used the fact that 1 and 2 are the SMALLEST to deduct that they MUST be assigned to vertices, not edges; and we’ve used the fact that 11 is the LARGEST to deduct that they must be assigned to an edge.
- Also see how we have used some symmetry to reduce the situation we need to consider.
What is the lesson we learned? Try – Think – Try again where we have a better chance. Use thinking / logic reasoning as a guidance so the trial can efficiently leads to result.
